jilovin.blogg.se

1. #TABULAR METHOD INTEGRATION BY PARTS PROOF HOW TO#
2. #TABULAR METHOD INTEGRATION BY PARTS PROOF MOVIE#

I try to prove it myself by going through each step. I see textbooks or web sites prove the method, but most of the times, they either do not explain each step or just skip with (.). We can also take u log(x) and dv x: Z log(x) x dx log(x) x2 2 Z 1 x x2 2 dx which is log(x)x22 x 4. I am learning repeated integration with the Tabular method.

#TABULAR METHOD INTEGRATION BY PARTS PROOF HOW TO#

Yes the methods of integration and the methods of differentiation are the same as integration is nothing but the reverse process of differentiation. Since we know from the previous problem how to integrate log we could proceed by taking x u. Are Methods of Integration Same as the Methods of Anti-differentiation? We use methods of integration when the function to be integrated is complex and hence simplify the function into simple forms whose integration is known. The most commonly used Integration methods are Integration by Parts, Method of Integration Using Partial Fractions, u-substitution method, Integration by Decomposition, and Reverse Chain Rule. What are the Most Commonly Used Integration Methods?

#TABULAR METHOD INTEGRATION BY PARTS PROOF MOVIE#

It has been called Tic-Tac-Toe in the movie Stand and deliver. This is why a tabular integration by parts method is so powerful. Keeping the order of the signs can be daunt-ing. This method of integration can be used if a function and its derivative are a part of the entire function to be integrated. Integration by parts can bog you down if you do it sev-eral times. Reverse Chain Rule Method of Integration is the counterpart of the Chai Rule of Differentiation and it is the exact reverse of the chain rule method. Which Method of Integration is the Counterpart of the Chain Rule of Differentiation? There are many methods of integration that we use but the most common ones are 5, namely Integration by Parts, Method of Integration Using Partial Fractions, Integration by Substitution Method, Integration by Decomposition, and Reverse Chain Rule. How many Types of Methods of Integration are there? Integration Using Trigonometric Identities.Method of Integration Using Partial Fractions.Given below is the list of the different methods of integration that are useful in simplifying integration problems: Differentiation and integration formulaįAQs on Methods of Integration What are the Methods of Integration in Calculus?.(a) Use integration by parts to prove the reduction formula Z (lnx)n dx x(lnx)n n Z (lnx)n1 dx (b) Evaluate R (lnx)3 dx 2. (a) R xcosxdx (b) R lnxdx (c) R x2e2x dx (d) R ex sin2xdx (e) Z lnx x dx Additional Problems 1. Add the constant of integration always after determining the integral of the function. The table above and the integration by parts formula will be helpful.It’s not always clear which is the best choice for u.

There are two parts to this function: (x 3 + 2x 1) and cos (4x).

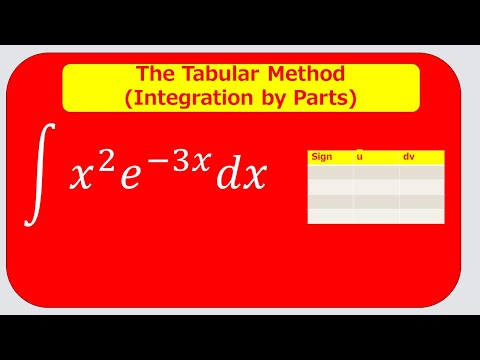
Please note that if we change the variable of integration, then it has to be changed throughout the integral. proof for tabular method, which is a generalization of the integration by parts. We differentiate f(x) = u which implies f'(x) dx = du ⇒ dx = du/h(u), where h(u) = f'(x) using f(x) = u-substitution. Then we can substitute the f(x) with another variable by assuming f(x) = u. For instance, we have integration of the form ∫g(f(x)) dx. This method enables us to change the variable of integration to simplify the function. The essential process of the above formula can be summarized in a table the resulting method is called 'tabular integration' and was featured in the film Stand and Deliver (1988). The substitution method is also commonly called the u-substitution method of integration. I came to know about the "Tabular Method" of integration by parts when I was looking for efficient ways of solving integrals involving the application of multiple times of integration by parts.Integration by Substitution Method of Integration